Answer:
Option C
Explanation:
Plan (i) Direction ratios of a line joining two points $(x_{1},y_{1},z_{1})$ and $(x_{2},y_{2},z_{2})$ are
$x_{2}-x_{1},y_{2}-y_{1},z_{2}-z_{1}$
(ii) If the two lines with direction ratios a1,b1, c1 ; a2,b2,c2 are perpendicular , then
a1a2+b1b2+c1c2=0
Line L1 given by y=x ;z=1 can be expressed
$L_{1}:\frac{x}{1}=\frac{y}{1}=\frac{z-1}{0}$
$\frac{x}{1}=\frac{y}{1}=\frac{z-1}{0}=\alpha$
$\Rightarrow$ $x=\alpha,y=\alpha,z=1$
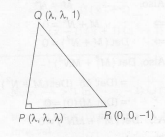
Let the coordinates od Q on L1 be $(\alpha,\alpha,1)$
Line L2 Given by y=-x , z=-1 can be expressed as
$L_{2}:\frac{x}{1}=\frac{y}{-1}=\frac{z+1}{0}$
$\frac{x}{1}=\frac{y}{-1}=\frac{z+1}{0}=\beta$ (say)
$\Rightarrow$ $x=\beta,y=-\beta,z=-1$
Let the coordinates of R on L2 be ($ \beta$, -$\beta$, -1)
Direction ratios of PQ are $\lambda-\alpha,\lambda-\alpha,\lambda-1$
Now PQ $\bot$ L1
$\therefore$ $1 (\lambda-\alpha)+1(\lambda-\alpha)+0.(\lambda-1)=0$
$\Rightarrow$ $ \lambda=\alpha$
$\therefore$ Q ( $\lambda$, $\lambda$,1)
direction ratio of PR are
$\lambda-\beta,\lambda+\beta,\lambda+1$
Now, PR $\bot$ L2
$\therefore$ $ 1(\lambda-\beta)+(-1)(\lambda+\beta)+0(\lambda+1)=0$
$\lambda-\beta-\lambda-\beta=0$
$\Rightarrow$ $ \beta=0$
$\therefore$ R(0,0,-1)
Now , as $\angle QPR= 90^{0}$
[ as a1a2+b1b2+c1c2 =0,
if two lines with DR'S a1,b1,c1:a2:b2:c2 are perpendicular)
$\therefore$ $(\lambda-\lambda)(\lambda-0)+(\lambda-\lambda)(\lambda-0)+(\lambda-1)((\lambda+1)=0$
$\Rightarrow$ $ (\lambda-1)(\lambda+1)=0$
$\Rightarrow$ $\lambda=1 $ or $\lambda=-1 $
$\lambda=1 $ , rejected as P and Q are different points
$\Rightarrow$ $ \lambda$=-1