JEE 2012 :: General Questions :: Physics

• General Questions - Physics
• General Questions - Chemistry
• General Questions - Mathematics

A mixture of 2 moles of helium gas (atomic mass = 4 amu) and 1 mole of  argon gas (atomic mass=40amu) is kept at 300K in a container. The ratio of the rms speeds $\left(\frac{v_{rms}(helium)}{v_{rms}(argon)}\right)is$

Answer:

Explanation:

$ v_{rms}=\sqrt{\frac{3RT}{M}} $  or $V_{rms}\propto \frac{1}{\sqrt{M}}$

$\therefore$   $ \frac{( v_{rms})_{He}}{( v_{rms})_{Ar}}=\sqrt{\frac{M_{Ar}}{M_{He}}}=\sqrt{\frac{40}{4}}=\sqrt{10}\approx3.16$

A small mass m is attached to a massless string whose other end is fixed at P as shown in the figure. The mass is undergoing circular motion in the x-y plane with centre at O  and constant angular speed $\omega$.If the angular momentum  of the system, calculated  about O and P  are denoted  by $L_{o}$ and $L_{p}$ respectively, then 

Answer:

Explanation:

Angular momentum of a particle about a
point is given by :

$L = r \times p =m (r \times v)$

 For $L_{0}$

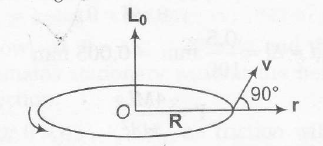
|L|=$(mvr \sin \theta)=m(R \omega)(R) \sin 90^{0}$

 =$mR^{2} \omega$= constant 

Direction of $L_{0}$ is always upwards , Therefore  , complete $L_{0}$  is constant , both  in magnitude as well as direction

For $L_{p}$

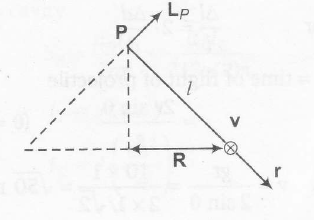
$|L_{p}|=(mvr \sin \theta)$

                     =$(m)(R\omega)(l) \sin90^{0}$

  =$(mRl \omega)$

magnitude  of $L_{p}$ will remain constant but  direction of $L_{p}$ keeps on changing 

A biconvex lens is formed with two thin plano-convex lenses as shown in the figure. The Refractive index n of the first lens is 1.5 and that of the second lens is 1.2. Both the curved surfaces are of the same radius of curvature R=74 cm. For this bi-convex lens, for an object distance of 40 cm, the image distance will be

Answer:

Explanation:

 Using the lens formula

$\frac{1}{v}-\frac{1}{u}=\frac{1}{f}=\frac{1}{f_{1}}+\frac{1}{f_{2}}$

 or   $\frac{1}{v}=\frac{1}{u}+\frac{1}{f_{1}}+\frac{1}{f_{2}}$

  = $\frac{1}{u}+(n_{1}-1)\left(\frac{1}{R_{1}}-\frac{1}{R_{2}}\right)$

 Substiuting the value , we get

 $\frac{1}{v}=\frac{1}{-40}+(1.5-1)\left(\frac{1}{14}-\frac{1}{\infty}\right)+$

$ (1.2-1)\left(\frac{1}{\infty}-\frac{1}{-14}\right)$

 Solving this equation , we get

 v=+40 cm

Two large vertical and parallel metal plates having a  separation of 1 cm are connected to a DC voltage source of potential difference  X. A proton is released at rest midway between the two plates. It is found to move at $45^{0}$ to the vertical just after release. Then X is nearly

Answer:

Explanation:

net force  is at $45^{0}$  from vertical

$\therefore$   qE=mg

or   $\frac{qX}{d}=mg$      ($\because E=\frac{X}{d}$)

 or      $X= \frac{mgd}{q}$

  =  $\frac{(1.67 \times 10^{-27})(9.8)(10^{-2})}{(1.6 \times 10^{-19})}$

    =$1 \times 10^{-9} V$

Young's double-slit experiment is carried out by using green,  red and blue light, one colour at a time. The fringe widths  recorded are $\beta_{G},\beta_{R}$ and $\beta_{B}$ respectively, then 

Answer:

Explanation:

Fringe  width $\beta= \frac{\lambda D}{d}$

or   $\beta \propto \lambda$

Now, $\lambda_{R} > \lambda_{G}  > \lambda_{B}$

$\therefore$   $  \beta_{R}$ > $\beta_{G}$>  $\beta_{B}$

• General Questions - Physics
• General Questions - Chemistry
• General Questions - Mathematics