Answer:
Option A
Explanation:
When dielectric slab of dielectric constant
K= $\frac{3}{2} $ is inserted between the plates of C2,
Its new capacitance (C2') becomes
C2'= $\frac{3}{2}$ C
Equivalent capacitance of C2' and C3 is
$C_{eq}=C_2'+C_{3}=\frac{3}{2}C+C=\frac{5C}{2}$
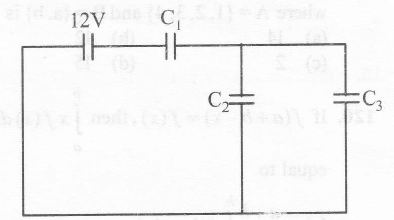
Now , Ceq and C1 are in series . Therefore , their equivalent capacitancce is
$C_{eq}=\frac{C_{eq}\times C_{1}}{C_{eq}+ C_{1}}=\frac{\frac{5C}{2}\times C}{\frac{5C}{2}+C}$
$=\frac{5C^{2}}{7C}=\frac{5C}{7}$