JEE 2016 :: Advanced 2016 :: Mathematics 2016

• Advanced 2016 - Physics 2016
• Advanced 2016 - Chemistry 2016
• Advanced 2016 - Mathematics 2016

 Football teams  $T_{1}$ and $T_{2}$ have to play two games against each other. It is assumed that the outcomes of the two games are independent. The probabilities of $T_{1}$ winning, drawing and losing a game against $T_{2}$ are  $\frac{1}{2},\frac{1}{6}and \frac{1}{3}$ ,respectively. Each team gets 3 points for a win, 1 point for a draw and 0 point for a loss in a game. Let X and Y  denote the total points  scored by teams $T_{1}$ and $T_{2}$, respectively, after two games

P(X=Y) is

Answer:

Explanation:

P[X=Y]=P(draw).P(draw)+ P( $T_{1}$ win )  P ( $T_{2}$ win)+ P(  $T_{2}$ win).P( $T_{1}$ win)

         $=(\frac{1}{6}\times \frac{1}{6})+ (\frac{1}{2}\times\frac{1}{3})+ (\frac{1}{3}\times\frac{1}{2})=\frac{13}{36}$

 Football teams  $T_{1}$ and $T_{2}$ have to play two games against each other. It is assumed that the outcomes of the two games are independent. The probabilities of $T_{1}$ winning, drawing and losing a game against $T_{2}$ are  $\frac{1}{2},\frac{1}{6}and \frac{1}{3}$ ,respectively. Each team gets 3 points for a win, 1 point for a draw and 0 points for a loss in a game. Let X and Y  denote the total points  scored by teams $T_{1}$ and $T_{2}$., respectively, after two games

   P(X>Y) is

Answer:

Explanation:

Here, P(X>Y) = P($T_{1}$ win) P($T_{1}$  win) +P( $T_{1}$  win )P ( draw) +P (draw) P ($T_{1}$  win)

                  =   $(\frac{1}{2}\times\frac{1}{2})+(\frac{1}{2}\times\frac{1}{6})+(\frac{1}{6}\times\frac{1}{2})=\frac{5}{12}$

Let  $a,\lambda,\mu \epsilon R$ . Consider the system of linear equation ax+2y=λ and 3x-2y= μ . Which of the following statement(s) is (are ) correct?

Answer:

Explanation:

Here, ax+2y=λ

and 3x-2y=μ

For a=-3 , above equations will be parallel  or coincident, ie, parallel for $\lambda+\mu\neq0$ and coincident. If $\lambda+\mu=0$ and if a≠ -3, equations are intersecting , i.e, unique solution

Let a,b ε R and $a^{2}+b^{2}\neq 0$ . Suppose  $S=( z\epsilon C: z=\frac{1}{a+ibt},t\epsilon R, t\neq0)$  where$i=\sqrt{-1}$ . If z=x+iy and z ε S, then (x,y) lies on

Answer:

Explanation:

Here,  $x+iy=\frac{1}{a+ibt}\times \frac{a-ibt}{a-ibt}$

$\therefore$    $x+iy=\frac{a-ibt}{a^{2}+b^{2}t^{2}}$

                    Let                     a≠ 0,b≠ 0

$\therefore$  $x=\frac{a}{a^{2}+b^{2}t^{2}}$ and    $y=\frac{-bt}{a^{2}+b^{2}t^{2}}$

$\Rightarrow$     $\frac{y}{x}=\frac{-bt}{a}\Rightarrow t=\frac{ay}{bx}$

On putting  $x=\frac{a}{a^{2}+b^{2}t^{2}},$ we get

                 $x(a^{2}+b^{2}.\frac{a^{2}y^{2}}{b^{2}x^{2}})=a$

                    $\Rightarrow a^{2}(x^{2}+y^{2})=ax$

                    or    $x^{2}+y^{2}-\frac{x}{a}=0$     .........(i)

             or         $(x-\frac{1}{2a})^{2}+y^{2}=\frac{1}{4a^{2}}$

  $\therefore$ Option (a) is correct.

      For a≠ 0, b=0

$x+iy=\frac{1}{a}\Rightarrow x=\frac{1}{a},y=0$

$\Rightarrow$ z lies on X-axis

$\therefore$    Option (c) is correct.

For a=0, b≠ 0,

$x+iy=\frac{1}{ibt}$

$\Rightarrow$    x=0, $y=-\frac{1}{bt}$

$\Rightarrow$  z lies on Y-axis

$\therefore$  Option (d) is correct

Let P be the point on the parabola  $y^{2}=4x$ , which is at the shortest distance from the centre S of the circle .$x^{2}+y^{2}-4x+16y+64=0$. Let Q be the point on the circle dividing the line segment  SP internally. Then, 

Answer:

Explanation:

Tangent  to $y^{2}=4x$  at  $(t^{2},2t)$ is 

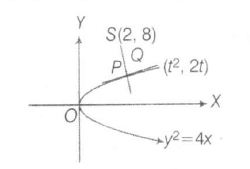
$y(2t)=2(x+t^{2})$

$\Rightarrow$              $yt=x+t^{2}$          ...........(i)

 Equation of normal  at $P(t^{2},2t)$  is

   $y+tx=2t+t^{3}$

 since, normal at P passes through centre of circle S (2,8).

$\therefore  8+2t=2t+t^{3}\Rightarrow t=2, i.e, P(4,4)$

 [Since, shortest distance between two curves lie along their common normal and the common  normal will pass through the centre of circle ]

$\therefore  SP=\sqrt{(4-2)^{2}+(4-8)^{2}}=2\sqrt{5}$

  $\therefore$ Option (a) is correct.

 Also, SQ=2

$\therefore   PQ=SP-SQ=2\sqrt{5}-2$

Thus,   $\frac{SQ}{QP}=\frac{1}{\sqrt{5}-1}=\frac{\sqrt{5}+1}{4}$

    $\therefore$  Option (b) is incorrect.

 Now, x- intercept of normal is 

                    $x=2+2^{2}=6$

 $\therefore$  Option (c) is correct.

  Slope of tangent= $\frac{1}{t}=\frac{1}{2}$

$\therefore$  Option (d) is incorrect

• Advanced 2016 - Physics 2016
• Advanced 2016 - Chemistry 2016
• Advanced 2016 - Mathematics 2016