Discussion Forum

A signal which can be green or red with probability 4/5 and 1/ 5 respectively, is received by station A and then transmitted to station B. The probability of each station receiving the signal correctly is 3/4. If the signal received at station B is given, then the probability that the original signal is green, is

Answer:

Explanation:

From the tree diagram, it follows that

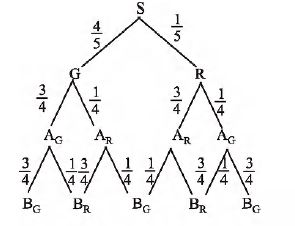
$P(B_{G}) = \frac{46}{80}$, $P(G) = \frac{4}{5}$

$P(B_{G}/G) = \frac{10}{16}$ = $\frac{5}{8}$

$\therefore P(B_{G}\cap G) = \frac{5}{8}\times\frac{4}{5}$ = $\frac{1}{2}$

$\left[\because P(B_{G}\cap G) = P\left(\frac{B_{G}}{G}\times P(G)\right)\right]$

Now , P(G/B_G)

$\frac{P(B_{G}\cap G)}{P(B_{G})}=\frac{1}{2}\times\frac{80}{40} = \frac{20}{23}$