Discussion Forum



1)

A current I flows in the anticlockwise direction through a square loop of side a lying in the xoy plane with its centre at the origin. The magnetic induction at the centre of the square loop is 


A) $\frac{2\sqrt{2}\mu_{0}I}{\pi a}\hat{e}_{x}$

B) $\frac{2\sqrt{2}\mu_{0}I}{\pi a}\hat{e}_{z}$

C) $\frac{2\sqrt{2}\mu_{0}I}{\pi a^{2}}\hat{e}_{z}$

D) $\frac{2\sqrt{2}\mu_{0}I}{\pi a^{2}}\hat{e}_{x}$

Answer:

Option B

Explanation:

 Field due to one side of loop at O= $\frac{\mu_{0}I}{4\pi (\frac{a}{2})}(2\sin 45^{0})$

 Field at O due to all four sides is along untill vector  $\hat{e}_{x}$

175202160_p5.JPG

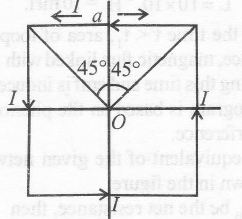
$\therefore$   Total field

 $=4.\frac{\mu_{0}I}{4\pi(\frac{a}{2})}(2\sin 45^{0})= \frac{2\sqrt{2}\mu_{0}I}{\pi a}$