Answer:
Option A
Explanation:
Suppose that both the balls meet at a distance h from ball -1 after time t from the start as shown in the figure,
For downward motion of ball-1, from second equation of the motion
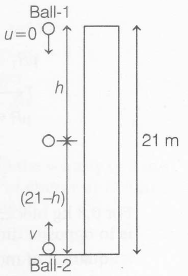
$h= ut+ \frac{1}{2} gt^{2}$ ......(i)
h= $0 \times t + \frac {1}{2} \times 10 t^{2}$
[ $\because u=0$]
h= $5 t^{2}$ ..........(ii)
For upward motion of ball -2 , from second equation of the motion.
$h= vt- \frac{1}{2}g t^{2}$ ........(iii)
Given, speed of ball -2 , v=14 m/s , acceleration due to gravity ,$ g=10 m/s^{2}$
Substituting these values in Eq.(iii) , we ger
$21-h=14 t -\frac{1}{2} \times 10 t^{2}$
$\Rightarrow$ $21-h=14t-5t^{2}$ ............(iv)
Adding Eq.(ii) and (iv) , we get
21=14 t
$\Rightarrow$ $ t= \frac{3}{2}s =1.5 s$
$\therefore$ Eq.(ii) , we get
h= $ 5 \times (1.5)^{2}$= 11.25 m
= $\frac{45}{4}$m
Therefore , the ball-1 will have dropped $\frac{45}{4}$ m when it passes ball-2