Discussion Forum



1)

A particle  of mass $m_{1}$ moving along X-axis collides with a stationary particle  of mass $m_{2}$ and deviates by an angle $30^{0}$ to the X-axis as shown inthe figure.If the percentage change in kinetic energy of the combined system of these two particles reduce by 50% , then the ratio  of the mass $\frac{m_{2}}{m_{1}}$ is 

2772021262_m4.PNG


A) 8

B) 6

C) $\frac{8}{7}$

D) $\frac{1}{6}$

Answer:

Option A

Explanation:

287202145_m5.PNG

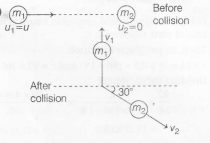
Momentum is conserved in both x and y directions separately

$\Rightarrow$    $m_{1}u=m_{2}v_{2}\cos 30^{0}$ ........(i)

and   $m_{1}v_{1}=m_{2}v_{2}\sin 30^{0}$  ........(ii)

 Also  , loss of K.E=50%

$\Rightarrow$     $50=\frac{\frac{1}{2}m_{1}u^{2}-\frac{1}{2}m_{1}v_{1}^{2}-\frac{1}{2}m_{2}v_{2}^{2}}{\frac{1}{2}m_{1}u^{2}}$ .......(iii)

 Solving eqs.(i), (ii) and (iii) , we get

 $\frac{m_{2}}{m_{1}}=8$