Answer:
Option D
Explanation:
Let Q(h,k) be the mid-point of the line joining the focus F(a,0) and variable point p(x0,y0).
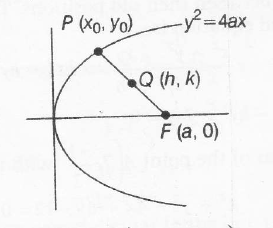
∴ (h,k)=(x0+a2,y02)
⇒ h=x0+a2 and k=y02
⇒ x0=2h−a and y0=2k
Since P(x0,y0) lies on parabola y2=4ax
∴ y20=4ax0⇒(2k)2=4a(2h−a)
⇒ 4k2=4a(2h−a)⇒k2=2a(h−a2)
Which is equation of parabola
∴ y2=2a(x−a2)
∴ Equation of directrix is given by
x−a2=−a2⇒x=0