Discussion Forum



1)

A positive charge Q is placed on a conducting spherical shell with inner radius $R_{1}$, and outer radius $R_{2}$, A particle with charge q is placed at the centre of the spherical cavity. The magnitude of the electric field at a point in the cavity, a distance r from the centre is 


A) zero

B) $\frac{Q}{4 \pi \epsilon_{0} r^{2}}$

C) $\frac{q}{4 \pi \epsilon_{0} r^{2}}$

D) $\frac{(q+Q)}{4 \pi \epsilon_{0} r^{2}}$

Answer:

Option B

Explanation:

1082021752_m8.PNG

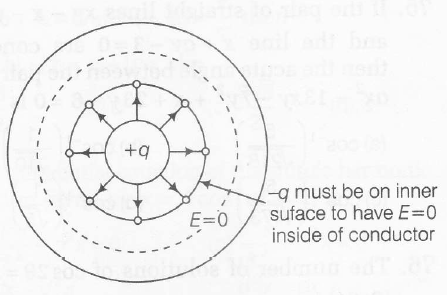
 Total charge on shell=+Q

 here, total charge on shell  is +Q . so the (-Q) is on the inner surface of shell.

 Hence, electric field inside the conductor =0

 according the Gauss's law

$\oint Eds=\frac{Q}{\epsilon_{0}}$

$E\oint ds=\frac{Q}{\epsilon_{0}}$

$E(4 \pi r^{2})=\frac{Q}{\epsilon_{0}}$

Electric field,  E=$\frac{Q}{4 \pi  \epsilon_{0} r^{2}}$