Discussion Forum



1)

 if $W_{1}$, $W_{2}$ and $W_{3}$ represent the work done in moving a particle from A to B  along three different paths 1,2 and 3 (as shown in fig) in the gravitational field of the point mass 'm'. Find  the correct relation between' $W_{1}$','$W_{2}$' and '$W_{3}$'

762021348_c3.JPG


A) $W_{1}$ < $W_{3} $ < $W_{2}$

B) $W_{1}$ < $W_{2} $ < $W_{3}$

C) $W_{1}$ = $W_{2} $ = $W_{3}$

D) $W_{1}$ > $W_{3} $ > $W_{2}$

Answer:

Option C

Explanation:

 Gravitational force is a conservative force. As we know that the work done by a conservative force is independent of the path followed and depends only on the endpoints of the motion. Since in the given pattern $W_{1}$,  $W_{2} $   and $W_{3}$ have  the  same  endpoint A and B as shown  in the figure below,

   762021321_c6.JPG

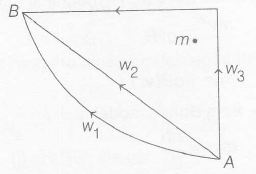
So, the correct relation between $W_{1}$  =  $W_{2} $   =  $W_{3}$