Discussion Forum

 Which of the following combinations of 7 identical capacitors each of 2$\mu$ F gives a resultant capacitance of 10/11 $\mu$ F?

Answer:

Explanation:

 Key Idea

       For n capacitors  i.e, C₁, C₂,.......C_n  their equivalent capacitance  , when connected in

  (a) series is given  as,  $\frac{1}{C_{s}}=\frac{1}{C_{1}}+\frac{1}{C_{2}}+....$

and (b) parallel is given as,   $C_{p}=C_{1}+C_{2}+....$

 In the given problem, we will check the net capacitance in each option and compare it with the desired capacitance mentioned in it.

   (a)   3 capacitors are in parallel and 4 capapcitors are connected in series, i.e,

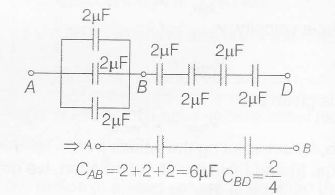
 where, C_AB is the equivalent capacitance  of parallel branches between A and C and  C_BD is equivalent capacitance of series capacitors  between B and D , So , equivalent capacitance

$C_{eq}=\frac{C_{AB}.C_{BD}}{C_{AB}+C_{BD}}=\frac{6\times\frac{2}{4}}{6 +\frac{2}{4}}=\frac{6}{13}\mu F$

 Similarly,

 (b) 2 capacitors are in parallel and 5 capacitors are connect in series i.e,

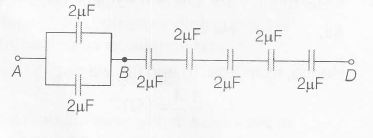
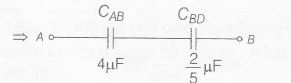
 So, equivalent capacitance,

  $C_{eq}==\frac{4\times\frac{2}{5}}{4 +\frac{2}{5}}=\frac{4}{11}\mu F$

 (c)   4 capacitors are in parallel and 3 capacitors are connect in series  i.e,

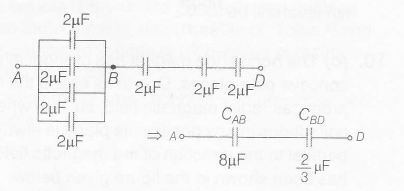
 So, equivalent capacitance,

$C_{eq}=\frac{8\times\frac{2}{3}}{8 +\frac{2}{3}}=\frac{16}{26}=\frac{8}{13}\mu F$

 (d)    5 capacitors are in parallel and 2 capacitors are connect in series i.e,

 So, equivalent capacitance

$C_{eq}=\frac{10\times1}{10 +1}==\frac{10}{11}\mu F$

 So, the correct option is (d)