Answer:
Option D
Explanation:
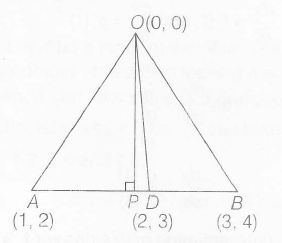
Given , O(0,0) , A(1,2) and B(3,4) be the vertices of $\triangle $ OAB
Let OP and OD be the altitude and median of $\triangle$ OAB, respectively
$\therefore$ Coordinates of D= Mid of AB.= $\left(\frac{1+3}{2},\frac{2+4}{2}\right)=\left(\frac{4}{2},\frac{6}{2}\right)=(2,3)$
Now, ewquation of OD is (y-0)= $\left(\frac{3-0}{2-0}\right)(x-0)$
$\left[\because y-y_{1}=\left(\frac{y_{2}-y_{1}}{x_{2}-x_{1}}(x-x_{1}\right)\right]$
$\Rightarrow$ $y=\frac{3}{2}x\Rightarrow2y=3x\Rightarrow3x-2y=0$
Slope of Op = -1/ Slope of AB $[\because OP\bot AB]$
=$\frac{-1}{\left(\frac{3-1}{4-2}\right)}=\frac{-1}{\frac{2}{2}}=-1$
$\therefore$ Equation of Op is (y-0) =-1(x-0)
$[\because y-y_{1}=slope (x-x_{1})]$
$\Rightarrow$ $y=-x\Rightarrow x+y=0$
Now, joint equation of OP and OD
(x+y)(3x-2y)=0
$\Rightarrow$ 3x2-2xy+3xy-2y2=0
$\Rightarrow$ $3x^{2}+xy-2y^{2}=0$