Discussion Forum

The angular width of the central maximum in a single slit diffraction pattern is 60° .The width of the slit is 1μm. The slit illuminated by monochromatic plane waves. If another slit of same width is made near it, Young's fringes can be observed on a screen placed at a disance 50cm from the slits. If the observed fringe width is 1 cm, what is slit seperation distance? (i.e. distance between the centres of each slit).

Answer:

Explanation:

Angular width of differaction pattern=60°

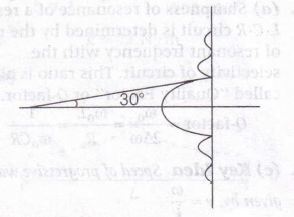
For first minima,

$\frac{a}{2}\sin\theta=\frac{\lambda}{2}$, [ here,a=10^-6m, θ=30°]

$\Rightarrow\lambda=10^{-6}\times \sin30^{0}\Rightarrow\lambda=\frac{10^{-6}}{2}m$

Now, in case of interference caused by bringing second slit,

$\therefore$    Fringe width, $\beta=\frac{\lambda D}{d}$

      [ here, 

$\lambda =\frac{10^{-6}}{2}m , \beta =1cm=\frac{1}{100}m,
d=?,and D=50cm=\frac{50}{100}m$ ]

So, $d=\frac{\lambda D}{\beta}=\frac{10^{-6}\times 50}{2\times \frac{1}{100}\times 100}=25\times 10^{-6}m$

or $d=25\mu m$