Discussion Forum

Tangents are drawn to the hyperbola  4x²-y²=36 at  the points P and Q . If these tangents intersect at the point T ( 0,3), then the area ( in sq units ) of $\triangle PTQ$ is

Answer:

Explanation:

Tangents are drawn to the hyperbola 4x²-y²=36 at the point P and Q.

Tangent intersects at point T ( 0,3).

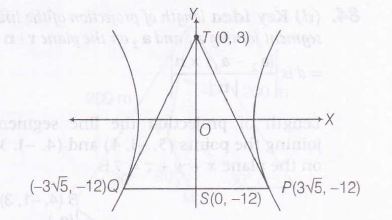
Clearly , PQ is chord of contact.

$\therefore$   Equation  of PQ is -3y=36

$\Rightarrow$   y=-12

Solving the curve 4x²-y²=36 and y=-12.

we get      x = $\pm 3\sqrt{5}$

Area of $\triangle PQT=\frac{1}{2}\times PQ \times ST= \frac{1}{2}(6\sqrt{5}\times 15)$

= $45\sqrt{5}$