Discussion Forum



1)

A diverging lens with a magnitude of focal length 25 cm is placed at a distance of 15 cm from a converging lens of the magnitude of focal length 20 cm. A  beam of parallel light falls on the diverging lens. The final image formed is


A) virtual and at a distance of 40cm from convergent lens

B) real and at a distance of 40cm from the divergent lens

C) rea; and at a distance of 6 cm from the convergent lens

D) real and at a distance of 40 cm from the convergent lens

Answer:

Option D

Explanation:

Focal length of the diverging lens is 25 cm.

   As the rays are coming parallel, so the image (I1) will be formed at the focus of diverging lens  i.e., at 25 cm towards left of diverging lens

 251020193_cmm.JPG

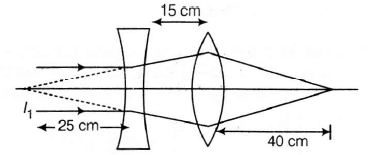
Now, the image (I1) will work as an object for a converging lens.

       For a converging lens, the distance of object u (i.e, the distance of I1)  = -(25+15)

                                                                                                              = -40 cm

                                                                      f  = 20 cm

$\therefore$       From lens 's formula  

  $\frac{1}{f}= \frac{1}{v}-\frac{1}{u}$

  $\frac{1}{20}= \frac{1}{v}-\frac{1}{-40}\Rightarrow \frac{1}{v}=\frac{1}{20}-\frac{1}{40}$

    $\frac{1}{v}= \frac{1}{90}\Rightarrow v =40 cm$

 v is positive so image will be real and will form at right side of converging lens at 40 cm.