Discussion Forum



1)

A  slender uniform rod of mass M and length l is pivoted at one end so that it can rotate in a vertical plane (see the figure). There is negligible friction at the pivot. The free end is held vertically above the pivot and then released. The angular acceleration of the rod when it makes an angle θ with the vertical is 

22102019269_ange.JPG


A) $\frac{2g}{3l}\sin\theta$

B) $\frac{3g}{2l}\cos\theta$

C) $\frac{2g}{3l}\cos\theta$

D) $\frac{3g}{2l}\sin\theta$

Answer:

Option D

Explanation:

As the rod rotates in the vertical plane so torque is acting on it, which is due to the vertical component of the weight of the rod.

22102019347_conditio.JPG

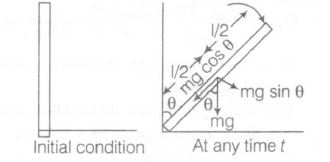
Now, Torque $\tau$ =force × perpendicular distance of the line of action of force from axis of rotation

                                           =  $mg\sin\theta \times\frac{1}{2}$

    Again , Torque $\tau= Iα$

Where, I = moment of interia =  $\frac{ml^{2}}{3}$

      [ Force and Torque frequency along axis of rotation passing through in end ]

$\alpha$ =  angular acceleration

  $\therefore$    $mg\sin\theta \times \frac{1}{2}=\frac{ml^{2}}{3}\alpha$

  $\therefore$    $\alpha   =\frac{3g\sin\theta}{2l}$