Answer:
Option A,C,D
Explanation:
Given a △XYZ ,where 2x=x+y+z
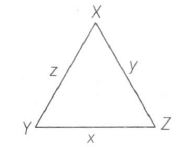
and s−x4=s−y3=s−z2
∴ \frac{s-x}{4}=\frac{s-y}{3}=\frac{s-z}{2}
= \frac{3x-(x+y+z)}{4+3+2}=\frac{s}{9}
or \frac{s-x}{4}=\frac{s-y}{3}=\frac{s-z}{2}=\frac{s}{9}=\lambda(let)
\Rightarrow s=9\lambda,s=4\lambda+x, s=3\lambda+y
and s=2\lambda+z
\therefore s=9\lambda,x=5\lambda, y=6\lambda, z=7\lambda
Now, \triangle= \sqrt{s(s-x)(s-y)(s-z)} [heron's formula]
= \sqrt{9\lambda.4\lambda.3\lambda.2\lambda}=6\sqrt{6}\lambda^{2} .........(i)
Also, \pi r^{2}=\frac{8\pi}{3}
\Rightarrow r^{3}=\frac{8}{3} .......(ii)
and R=\frac{xyz}{4\triangle}
=\frac{(5\lambda)(6\lambda)(7\lambda)}{4.6.\sqrt{6}\lambda^{2}}=\frac{35\lambda}{4\sqrt{6}} .......(iii)
Now, r^{2}=\frac{8}{3}=\frac{\triangle^{2}}{S^{2}}=\frac{216\lambda^{2}}{81\lambda^{2}}
\Rightarrow \frac{8}{3}=\frac{8}{3}\lambda^{2} [from Eq. (ii)]
\Rightarrow \lambda=1
(a) \triangle XYZ=6\sqrt{6}\lambda^{2}=6\sqrt{6}
\therefore Option (a) is correct.
(b) Radius of circumcircle
(R)=\frac{35}{4\sqrt{6}}\lambda=\frac{35}{4\sqrt{6}}
\therefore Option (b) is incorrect.
(c) Since, r= 4R\sin\frac{X}{2}.\sin\frac{Y}{2}.\sin\frac{Z}{2}
\Rightarrow\frac{2\sqrt{2}}{\sqrt{3}}=4.\frac{35}{4\sqrt{6}}\sin\frac{X}{2}\sin\frac{Y}{2}\sin\frac{Z}{2}
\Rightarrow\frac{4}{35}=\sin\frac{X}{2}\sin\frac{Y}{2}\sin\frac{Z}{2}
\therefore Option (c) is correct.
(d) \sin^{2}\left(\frac{X+Y}{2}\right)=\cos^{2}\left(\frac{Z}{2}\right) . as
\frac{X+Y}{2}=90^{0}-\frac{Z}{2}=\frac{s(s-z)}{xy}=\frac{9\times 2}{5\times 6}=\frac{3}{5}
\therefore Option (d) is correct
