Discussion Forum

 In a thin rectangular metallic strip, a constant current I flows along the positive x-direction, as shown in the figure. The length,  width and thickness of the strip are l, w and d respectively. A uniform magnetic field  B is applied on the strip along the positive y-direction. Due to this, the charge carriers experience a net deflection along the z-direction. This results in the accumulation of charge carries on the surface PQRS and the appearance of equal and opposite charges on the face opposite to PQRS. A potential difference along the z-direction is thus developed. Charge accumulation continues untill the magnetic force is balanced by the electric force. The current is assumed to be uniformly distributed on the cross-section of the strip and carried by electrons.

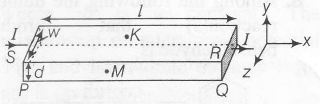
 Consider two different metallic strips (1  and 2)  of same dimensions (length l, width w and thickness d)  with carrier densities n₁  and n₂, respectively. Strip  1 is placed in magnetic field  B₁  and strip 2 is placed in magnetic field B₂, both along positive y- directions. Then V₁  and V₂ are the potential differences developed between K and M in strips  1 and 2, respectively. Assuming that the current I is the  same for both the strips, the correct options is/are

Answer:

Explanation:

   $V=\frac{BI}{ned}$

   $\Rightarrow$                  $\frac{V_{1}}{V_{2}}=\frac{B_{1}}{B_{2}}\times\frac{n_{2}}{n_{1}}$

  If  If $B_{1}=B_{2}$  and $n_{1}=2n_{2}$ , then $V_{2}=2V_{1}$

   If $B_{1}=2B_{2}$  and $n_{1}=n_{2}$ , then $V_{2}=0.5V_{1}$

$\therefore$   Correct answers are (a) and (c).