Discussion Forum

1)

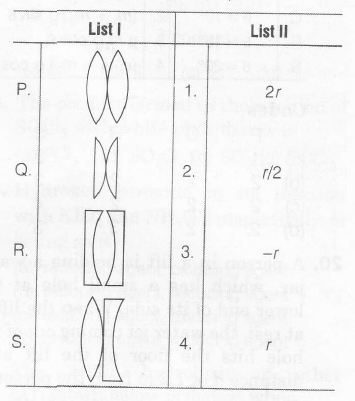
Four combinations of two thin lenses are given in List I. The radius of curvature of all curved surfaces is r and the refractive index of all the lenses is 1.5. Match lens combinations in List I with their focal length in List-II and select the correct answer using the code given below the lists

Answer:

Option B

Explanation:

(P)  $  \frac{1}{r}=\left(\frac{3}{2}-1\right)\left(\frac{1}{r}+\frac{1}{r}\right)=\frac{1}{r}$

          $\Rightarrow f=r$

           $\Rightarrow$           $\frac{1}{f_{eq}}=\frac{1}{f}+\frac{1}{f}=\frac{2}{r}$

            $\Rightarrow$    f_eq=\frac{r}{2}$

    

(Q)   $  \frac{1}{r}=\left(\frac{3}{2}-1\right)\left(\frac{1}{r}\right)\Rightarrow f=2r$

                $\Rightarrow$     $\frac{1}{f}+\frac{1}{f}=\frac{2}{f}= \frac{1}{r}$

                  $\Rightarrow$    f_eq=r

(R)     $  \frac{1}{f}=\left(\frac{3}{2}-1\right)\left(-\frac{1}{r}\right)\Rightarrow -\frac{1}{2r}$

                      $\Rightarrow$    f=-2r

          

$\Rightarrow$           $\frac{1}{f_{eq}}=\frac{1}{f}+\frac{1}{f}=\frac{2}{2r}$

    

$\Rightarrow$    f_eq=-r

 

(S)          

           $\frac{1}{f_{eq}}=\frac{1}{r}+\frac{1}{-2r}=\frac{1}{2r}$

       $\Rightarrow$    f_eq=2r