Discussion Forum

The figure shows a circular loop of radius   $\alpha$  with two long parallel wires (numbered 1 and 2)  all in the plane of the paper. The distance of each wire from the centre of the loop is d, The loop and the wires are carrying the same current I. The  current in the loop is in the counter-clockwise direction if seen from above

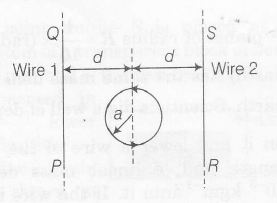
 When d≈a  but wires are not touching the loop, it is found that the net magnetic field on the axis of the loop is zero at a height h above the loop. In that case

Answer:

Explanation:

B_R =B due to ring

B₁ = B due to wire-1

B₂= B due to wire -2

 In magnitudes

     B₁  =B₂ =  $\frac{\mu_{0}l}{2\pi r}$

 Resultant of B₁ and B₂

$=2B_{1}\cos \theta =2\left(\frac{\mu_{0}l}{2\pi r}\right)\left(\frac{h}{r}\right)$

   = $\frac{\mu_{0}lh}{\pi r^{2}}$

   $B_{R}=\frac{\mu_{0}lR^{2}}{2(R^{2}+X^{2})^{3/2}}$

$=\frac{2\mu_{0}l\pi a^{2}}{4\pi r^{3}}$

   As , R=a ,x=h  and a²+h²=r²

 For zero magnetic field at P.

  $\frac{\mu_{0}lh}{\pi r^{2}}=\frac{2\mu_{0}l\pi a^{2}}{4\pi r^{3}}$

    $\Rightarrow$       $\pi a^{2}=2rh\Rightarrow$ h≈1.2 a