Discussion Forum

Let   $n_{1}$<$n_{2}$ <$n_{3}$<$n_{4}$  < $n_{5}$  be positive integers such that $n_{1}$+$n_{2}$+$n_{3}$+$n_{4}$+$n_{5}$=20. The numbers of such  distinct arrangements ( $n_{1}$,$n_{2}$,$n_{3}$,$n_{4}$,$n_{5}$)    is 

Answer:

Explanation:

Plan, Reducing the equation to a newer question, where sum of variables is less. Thus , find ing the number of arrangements  becomes easier

 As, $n_{1}\geq 1$ , $n_{2}\geq 2$, $n_{3}\geq 3$, $n_{4} \geq 4$,$n_{5}\geq 5$ 

 Let  n₁ -1= x₁ $\geq$ 0,  n₂-2 =x₂  $\geq$ 0, ....... n₅-5 =x₅  $\geq$ 0

New equation will be

x₁+1+x₂+2+.........x₅+5=20

    x₁+x₂+x₃+x₄+x₅

                    =20-15=5

  Now,    x₁  $\leq$  x₂ $\leq$ x₃   $\leq$ x₄   $\leq$ x₅

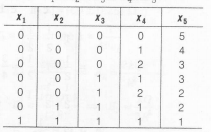
 So, 7 possible  cases will be there