Discussion Forum



1)

  The x- coordinate of the incentre of the triangle that has the coordinates of mid-points of its sides as (0,1),(1,1) and (1,0) is


A) $2+\sqrt{2}$

B) $2-\sqrt{2}$

C) $1+\sqrt{2}$

D) $1-+\sqrt{2}$

Answer:

Option B

Explanation:

 Given midpoints  of a triangle are (0,1),(1,1)  and (1,0) . Plotting these points on graph paper and make a triangle. 

 So the sides of a triangle will be 2,2 and  $ \sqrt{2^{2}+2^{2}}$  $i.e 2\sqrt{2}$

  542021220_m4.JPG

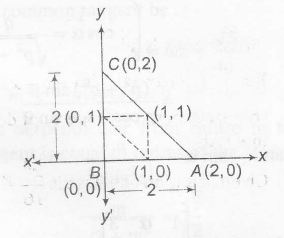
 x- coordinate of incentre

  =$\frac{2\times0+2\sqrt{2}.0+2.2}{2+2+2\sqrt{2}}$

  $=\frac{2}{2+\sqrt{2}}\times\frac{2-\sqrt{2}}{2-\sqrt{2}}=2-\sqrt{2}$