Answer:
Option A
Explanation:
We know that, the sum of three vectors of a triangle is zero.
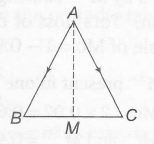
$\therefore$ AB+BC+CA=0
$\Rightarrow$ BC=AC-AB
$\Rightarrow$ $BM=\frac{AC-AB}{2}$
($\because$ M is a mid point of BC )
Also, AB+BM+MA=0
(By properties of a triangle)
$\Rightarrow $ $ AB+\frac{AC-AB}{2}=AM$
$\Rightarrow$ $ AM=\frac{AB+AC}{2}$
= $\frac{3\hat{i}+4\hat{k}+5\hat{i}-2\hat{k}+4\hat{k}}{2}$
= $4\hat{i}-\hat{j}+4\hat{k}$
$\Rightarrow$|AM|= $\sqrt{4^{2}+1^{2}+4^{2}}=\sqrt{33}$