Answer:
Option A,B
Explanation:
Concept lnvolved
Equation of tangent to x2a2−y2b2=1 is
y=mx±√a2m2−b2
Description of Situation If two straight
lines
a1x+b1y+c1=0
and ax2+b2y+c2=0 are identical
a1a2=b1b2=c1c2
sol., Equation of tangent , parallel to
y=2x-1
⇒ y=2x±√9(4)−4
∴ y=2x±√32....(i)
The equation of tangent at (x1,y1) is
xx19−yy14=1 ...(ii)
from Eqs(i) and (ii) , we get
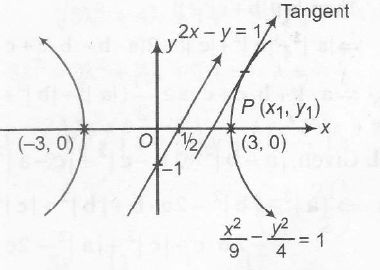
2x19=−1−y14=±√321
⇒ x1=−92√2andy1=−1√2
or x1=92√2 and y1=1√2