Discussion Forum



1)

A metal rod of length L and mass m is pivoted at one end. A thin disc of mass M and radius R (< L) is attached at its centre to the free end of the rod. Consider two ways the disc is attached. Case  A-the disc is not free to rotate about its centre and case B-the disc is free to rotate about its centre. The rod-disc system performs  SHM in a vertical plane after being released from the same displaced position.

Which of the following statement(s) is/are true?

28112021523_u7.PNG


A) Restoring torque in case A = Restoring torque in case B

B) Restoring torque in case A < Restoring torque in case B

C) Angular frequency for case A > Angular frequency for case B

D) Angular frequency for case A < Angular frequency for case B

Answer:

Option A,D

Explanation:

$\tau_{A}=\tau_{B}=mg \frac{L}{2} \sin \theta +MgL \sin \theta$

= Restoring torque about Point O.

In case A, a moment of inertia will be more. Hence, angular acceleration ($\alpha= \tau/I$) will be less. Therefore, the angular frequency will be less.

28112021244_h.PNG

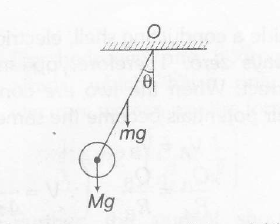
 $\therefore$ Correct options are (a) and (d)

Analysis of Question
The question is difficult from my point of view. Because this type of SHM is rarely taught in the class and questions of this type are not given in standard books