Answer:
Option A,C,D
Explanation:
Thermal resistance
$ R= \frac{l}{KA}$
$\therefore$ $R_{A}= \frac{ L}{(2K)(4L \omega)}=\frac{1}{ 8K \omega}$
(here , $\omega$= width)
$R_{B}= \frac{ 4L}{3K(L \omega)}= \frac{4}{ 3K \omega}$
$R_{C}= \frac{4L}{(4K)(2L \omega)}=\frac{1}{2K \omega}$
$R_{D}=\frac{4L}{(5K)(L \omega)}=\frac{4}{5 K \omega}$
$R_{E}= \frac{L}{(6K)(L \omega)}=\frac{1}{6 K \omega}$
$R_{A}:R_{B}:R_{C}:R_{D}:R_{E}$
=15:160:60:96:12
So, Let us write , $R_{A}=15 R$,$R_{B}=160 R$ etc and draw a simple electrical circuit as shown in figure.
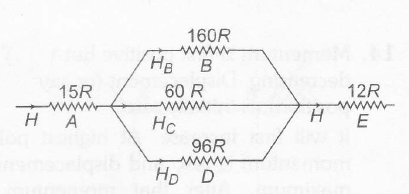
H= Heat current = Rate of heat flow
$H_{A}=H_{E}=H$ (let)
$\therefore$ option (a) is correct
in parallel current distributes in inverse ratio of resistance
$\therefore$ $H_{B}:H_{C}:H_{D}= \frac{1}{R_{B}}:\frac{1}{R_{C}}:\frac{1}{R_{D}}$
= $\frac{1}{160}:\frac{1}{60}:\frac{1}{96}$
=9:24-:15
$\therefore$ $H_{B}=\left(\frac{9}{9+24+15}\right)H= \frac{3}{16} H$
$H_{C}=\left(\frac{24}{9+24+15}\right)H= \frac{1}{2} H$
and $H_{D}=\left(\frac{15}{9+24+15}\right)H= \frac{5}{16} H$
$H_{C}=H_{B}+H_{D}$
$\therefore$ option (d) is correct
Temperature difference (let us call it T)= (Heat current ) x (Thermal resistance)
$T_{A}= H_{A} R_{A}= (H) (15 R) =15 HR$
$T_{B}=H_{B}R_{B}==\left(\frac{3}{16}H\right) (160 R)=30HR$
$T_{C}=H_{C}R_{C}==\left(\frac{1}{2}H\right) (60 R)=30HR$
$T_{D}=H_{D}R_{D}==\left(\frac{5}{16}H\right) (96 R)=30HR$
$T_{E}=H_{E}R_{E}=(H) (12 R)=12 HR$
Here, $T_{E}$ is minimum . Therefore option (c) is also correct
$\therefore$ Correct options are (a) (c) and (d)
Analysis of Question
(i) From calculation point of view, question is difficult otherwise the question is simple.
(ii) In heat transfer, questions are mainly asked from conduction and radiation topic.